Eric Weeks - personal pages - computer-generated pictures Cellular Automata on a QuasiCrystal

weeks/physics.emory.edu

Description

The information on this page is sketchy; for more detailed information on the algorithm, click here or the picture.

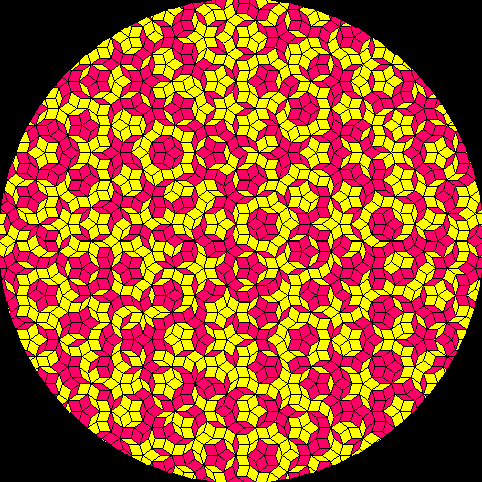
This picture was generated on a quasi-periodic tiling of the plane possessing 5-fold symmetry.

I used a depth-1 neighborhood (each tile considers what the four tiles touching it are like; the tiles must share an edge). The fat tiles are like sheep: they want to be like their neighbors. If they have 3 or 4 neighbors (not counting themselves) of one color, they switch to that color. The skinny tiles are contrary; probably they'll vote for Ross Perot. If they have 3 or 4 neighbors (not counting themselves) of one color, they switch to the opposite color.

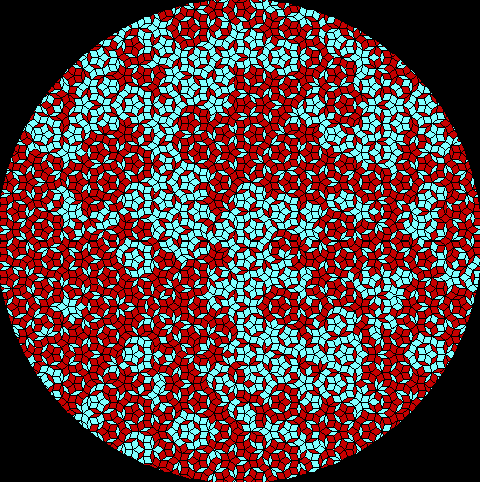
This was generated with a similar rule. The fat tiles switch color to agree with their neighbors if a simple majority of them are one color (counting themselves this time). The skinny tiles are still contrary, and they switch colors to disagree if a simple majority of neighbors are one color (counting themselves). In this case I'm using a depth-3 neighborhood.

What's a depth-3 neighborhood mean? I told you the information on this page is sketchy; click here to get more of the details on this algorithm.

Curious what the Earth would look like from space if we had a 3-party system world government? Click here for more information. Or, perhaps it's just a 3-party majority rule CA run on a quasi crystal ...

A simpler CA run on a quasicrystal.

Links...

• CLICK HERE FOR INFORMATION ABOUT SOFTWARE USED TO MAKE QUASICRYSTALS
• Software I've written -- some functional, some fun
• Research I do
• Various simple algorithms for making simple pictures
• Graphics techniques (some used to make these pictures)
• Questions? Comments? Send me email; email address below.