Practice Questions
These questions are designed to test your knowledge of evolutionary game theory, give you practice with problem solving, and prepare you for the type of questions that might appear on an exam. After answering each question, use the ✓ button to see the correct answer. (For best results, avoid the temptation to check the answer before giving the question your best shot!)
This set of questions refers to the four graphs shown here. Each graph represents change over time in an evolutionary game. The y-axis is the proportion of the population playing a strategy and the x-axis is time. If there are two curves on the same graph, it means that either curve is possible for the strategy.
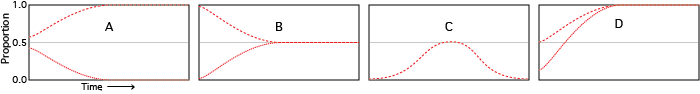
The questions ask you to choose the graph that best fits a situation; you may use a graph more than once or not at all.

The questions ask you to choose the graph that best fits a situation; you may use a graph more than once or not at all.
Even very mundane events can be modeled with game theory. For example, two people simultaneously approach a glass door from opposite sides. When the door is opened, it confers a benefit b to each person, but there’s also a cost c to the one who pushes or pulls the door open. If both people open the door simultaneously, they split the cost equally.