Random Close Packing

• "Random close packing of disks and spheres in confined geometries"
  KW Desmond & ER Weeks, Phys. Rev. E 80, 051305 (2009).
  • Abstract / PDF / journal / arXiv:0903.0864 / source code
• "Experimental study of random close packed colloidal particles"
  R Kurita & ER Weeks, Phys. Rev. E 82, 011403 (2010).
  • Abstract / PDF / journal / arXiv:1003.3965 / supplemental material (particle coordinates)
• "Incompressibility of polydisperse random close packed colloidal particles"
  R Kurita & ER Weeks, Phys. Rev. E 84, 030401(R) (2011).
  • Abstract / PDF / journal / arXiv:1107.1765
• "Influence of particle size distribution on random close packing"
  KW Desmond and ER Weeks, Phys. Rev. E. 90, 022204 (2014)
  • Abstract / PDF / journal / arXiv:1303.4627 / download our data
• "Random packing of rods in small containers"
  JO Freeman, S Peterson, C Cao, Y Wang, SV Franklin, & ER Weeks, Granular Matter 21, 84 (2019).
  • Abstract / PDF / journal / arXiv:1902.00565 / download our data
• Bounded distributions place limits on skewness and larger moments
  DJ Meer & ER Weeks, PLoS ONE 19, e0297862 (2024).
  • Abstract / PDF / journal / arXiv:2308.05006 / Appendix (PDF) / PubPeer comments / download our data (zip)
• "Circle radius distributions determine random close packing density"
  DJ Meer, I Galoustian, JG de Falco Manuel, and ER Weeks, Phys. Rev. E 109, 064905 (2024).
  • Abstract / PDF / journal / arXiv:2404.02316
• Ken Desmond's PhD dissertation -- chapter 3 in particular

Random close packing is the idea that particles often pack densely in jumbled random arrangements. This can be gumballs, beans, or rice in containers; sand at a beach; marbles in a bag. If all the particles are the same size, sometimes they pack less randomly: some of the gumballs in the image above are nestled into hexagonal structures (image credit: Eric).

Introduction As the list of papers above suggests, we've been interested in the question of how particles pack randomly for several years. This has been a mixture of experimental and computational work. A computational image is shown at right. The basic question we ask about random close packed structures is how densely they are packed: what is the volume fraction (phi) of the particles? That is, what fraction of the volume is solid particles, with the remainder being empty space between the particles? This is called by the Greek letter phi. For mixtures of spheres that are all the same size, the answer for the volume fraction of random close packing is known to be about 0.63 - 0.64. That is, just under two thirds of the volume is solid spheres. The exact number depends on how you do the experiment or simulation. Of course, one can ask more questions beyond just the volume fraction. What does it mean to pack particles "randomly"? What happens if the particles are different shapes, or mixtures of different sizes? In the picture above with gumballs, the container walls may be changing the packing and causing the hexagonal patterns some of the particles are making; how does a particle pack "randomly" against a wall? These are some of the questions we've investigated.

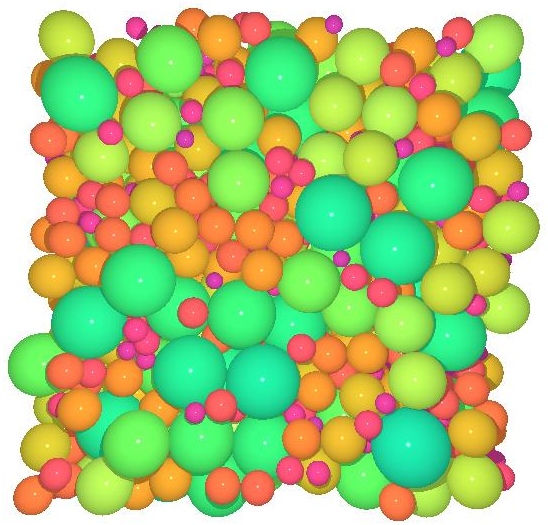A computer-generated random close packed configuration. The polydispersity (standard deviation of particle sizes divided by the mean size) is 0.4.

Computer algorithm We use a method adapted from Xu, Blawzdziewicz, and O'Hern, PRE 71, 061306 (2005). To computationally generate random close packing states, we start with tiny particles randomly scattered in the simulation box. If we want a mixture of sizes, we pick them appropriately. We then slowly grow all of the particles, keeping their ratios of sizes constant. (For example if we want a mixture of particles with a size ratio 1 : 1.4, at each step we might grow the particles by 0.1% each so that their size ratio stays constant. At each growth step, if the particles overlap, we treat them as soft particles that repel and move them apart. If the particles don't overlap, we let them move a small step randomly, which helps fill in the voids between particles. If at some point we can't move particles to prevent overlapping, then we shrink all of them a tiny bit to remove the overlap. Ultimately we find a close packed state where no particles are overlapping, but where we can't grow them any larger. As mentioned above, the final value of the volume fraction depends on the algorithm, so we don't claim our results are universal. For monodisperse spheres we find the random close packed volume fraction phi is 0.639. For a mixture of 2D circles with size ratio 1 : 1.4, we find phi = 0.841, which compares favorably with previously published results.

Click here for an animated GIF movie which shows our algorithm for making random close packing. The square box indicates the periodic boundary conditions: particles wrap around from one side of this box to the other.

Key Results

Confinement: If you try to pack particles in small containers, they pack less efficiently near the walls. Conceptually, there's a boundary layer of poorly packed particles near the wall. This finding suggests a way to extrapolate to infinite container size. Discussed in Desmond & Weeks, PRE 2009. This was done computationally, following the algorithm above but with rigid walls (click here for animation). This project was originally inspired by our interest in the colloidal glass transition studied in confinement. The image at left is from one of our simulations. The particles have a size ratio 1 : 1.4 and equal numbers of each size.

Rods: We were curious how these results would work with different shaped particles, and plastic rods were the easiest to get. We packed them in cylindrical containers of a variety of sizes, see photo at right. X-ray tomography showed that the boundary layers are about half the rod length in thickness. Overall, higher aspect ratio rods (longer and/or thinner rods) pack less densely, in agreement with prior experimental and computational work. The results are presented in Freeman et al, GM 2019. The photo at left shows the top third of one of our containers containing plastic rods; click to see larger image. (Image credit: Julian Freeman)

Random close packing of colloids: If colloidal particles are allowed to sediment in a jar, they can settled into a random close packed state. We were curious how similar this is to computer-generated random close packed states. We used confocal microscopy to image such a colloidal sample. By stitching together overlapping 3D images, we were able to observe half a million particles. Initially we thought our observations disproved hyperuniformity (Kurita & Weeks, PRE 2010) but then a subsequent reanalysis which took into account slight particle size variations showed that our sample is indeed hyperuniform (Kurita & Weeks, PRE 2011). Hyperuniformity is a particular statistical property of some random packings. In particular, the density varies from location to location within the sample because of the randomness of the packing, but these density fluctuations disappear more rapidly than might be expected as one considers larger and larger location chunks. The image at left shows 2 micron diameter colloidal PMMA particles, viewed by confocal microscopy. (Image credit: Rei Kurita)

Random close packing of mixtures of particle sizes: In most real samples, particles aren't all the same size. It is known that more polydisperse samples pack more densely; polydispersity is defined as the standard deviation of particle sizes divided by the mean particle size. A collection of particles of various sizes is characterized by the histogram of the particle sizes. Different samples can have the same mean size and polydispersity and yet have quite different histograms. We found that knowing the polydispersity and skewness is sufficient to determine the volume fraction with high accuracy. (Skewness is another statistical measure of data, related to how symmetric the histogram is.) Discussed in Desmond & Weeks, PRE 2014. The image at left is from one of our simulations of a mixture of two different sphere sizes.

RCP of mixtures of particle sizes in 2D: Our 2014 results were in 3D. We then studied the same question in 2D (Meer et al., 2024). As a side project, we realized that for probability distributions of positive quantities (such as circle sizes), there is a minimum possible value of the skewness (see Meer & Weeks, PLoS ONE 2024). For the picture at left, the large particles are colored according to psi6. Bluer colors indicate particles that are hexagonally ordered relative to their large particle neighbors, whereas green and orange particles are less hexagonally ordered. The small white particles are ignored in that calculation, but their presence helps randomize the larger particles to decrease the hexagonal ordering somewhat.

For fun

We also tried some 2D experiments for a while; one experimental image is below. We used laser-cut plastic rings of various sizes in a large one meter diameter 2D container. Photo from Isabela Galoustian and David Meer.